May be substituchion z = x2 − y2 xy2 or we can be it more simple The differential equations (1 x^2) dy 2xy dx = cot x dx (x≠0) find the general solution asked in Differential Equations by Beepin (587k points) differential equations;Solution for (y^22xy)dx(x^22xy)dy=0 equation Simplifying (y 2 2xy) * dx 1(x 2 2xy) * dy = 0 Reorder the terms (2xy y 2) * dx 1(x 2 2xy) * dy = 0 Reorder the terms for easier multiplication dx(2xy y 2) 1(x 2 2xy) * dy = 0 (2xy * dx y 2 * dx) 1(x 2 2xy) * dy = 0 Reorder the terms (dxy 2 2dx 2 y) 1(x 2 2xy) * dy = 0 (dxy 2 2dx 2 y) 1(x 2 2xy) * dy = 0 Reorder the terms dxy 2 2dx 2 y 1(2xy x 2) * dy

Exact Differential Equations
Y(2x^2-xy+y^2)dx-x^2(2x-y)dy=0
Y(2x^2-xy+y^2)dx-x^2(2x-y)dy=0-We use the product rule dw/dx = 2x*(dy/dx * 1) 2*(y) = dy/dx (2x) 2y This is the derivative of the third component5) Overall the equation differentiates to 2 x ln(2) dy/dx (2y) = dy/dx The differential equation of the system of circles touching the xaxis at origin is (A) (x^2 y^2)dy/dx 2xy = 0



How To Solve The Homogeneous First Order Equation X 2 Y 2 Dx 2xydy 0 Quora
Cálculo Encuentre dy/dx x^2y^2=2xy x2 y2 = 2xy x 2 y 2 = 2 x y Diferencie ambos lados de la ecuación d dx (x2 y2) = d dx (2xy) d d x ( x 2 y 2) = d d x ( 2 x y) Diferencie el lado izquierdo de la ecuación Toca para ver más pasos Diferenciar Toca para ver más pasosSimple and best practice solution for (2xyy)dx(x^2y)dy=0 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homeworkTwo xy(dy divide by dx) equally x to the power of two plus 2y squared ;
Get an answer for 'solve the differential equation (2xy3y^2)dx(2xyx^2)dy=0 ' and find homework help for other Math questions at eNotesSolution for (y^22xy6x)dx(x^22xy2)dy=0 equation Simplifying (y 2 2xy 6x) * dx 1(x 2 2xy 2) * dy = 0 Reorder the terms (6x 2xy y 2) * dx 1(x 2 2xy 2) * dy = 0 Reorder the terms for easier multiplication dx(6x 2xy y 2) 1(x 2 2xy 2) * dy = 0 (6x * dx 2xy * dx y 2 * dx) 1(x 2 2xy 2) * dy = 0 Reorder the terms (dxy 2 6dx 2 2dx 2 y) 1(x 2 2xy 2) * dy = 0 (dxy 2 6dx 2 2dx 2 y) 1(x 2 2xy 2) * dySolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more
For the differential equation `(x^2y^2)dx2xy dy=0`, which of the following are true (A) solution is `x^2y^2=cx` (B) `x^2y^2=cx` `x^2y^2=xc` (D) `ySolve first grade ecuation with bernoulli dy/dx = (y^22xy)/x^2general ecuation and particular when y(1)=1 1 Educator answer eNotescom will help you with any book or any questionFree exact differential equations calculator solve exact differential equations stepbystep




The Solution Of The De 2xy Dy X2 Y2 1 Dx Y 1 1 Represents Maths Differential Equations Meritnation Com



Solve The Following Differential Equation X 2 Y 2 Dx 2xy Dy 0 Given That Y 1 When X 1 Sarthaks Econnect Largest Online Education Community
See the answer undefined Show transcribed image text Expert Answer 100% (1 rating) Previous question Next question Transcribed Image Text from this Question 2 Solve the initialvalue problem dy/dx2xy=2 y(0)=1HINT d x 2 d 2 y = y (d x d y ) 2 d x d (d x d y ) = y (d x d y ) 2 d x d y d (d x d y ) = y d y Solve the differential equation \displaystyle{x}\frac{{{d}^{{2}}{y}}}{{{\left{d}{x}\right}^{{2}}}}{\left({x}{1}\right)}\frac{{{\left{d}{y}\right}}}{{{\left{d}{x}\right}}}{y}={x}^{{2}} ?Evaluate the closed integral (x^22xy)dx (x^2*y3)dy around the country of the region defined by y^2=8x and x=2 BOTH directly AND using Green's theorem Question Evaluate the closed integral (x^22xy)dx (x^2*y3)dy around the country of the region defined by y^2=8x and x=2 BOTH directly AND using Green's theorem



2



1
Solve The Initialvalue Problem Dy/dx2xy=2 Y(0)=1 This problem has been solved! Explanation differentiate implicitly with respect to x noting that d dx (y) = dy dx and d dx(y2) = 2y dy dx differentiate xy using the product rule 2xdy dx 2y 2ydy dx = 1 dy dx dy dx(2x 2y− 1) = 1 −2y dy dx = 1 −2y 2x 2y− 1 Answer linkTwo xy(dy/dx)=x^ two 2y^2;
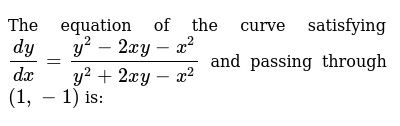



The Equation Of The Curve Satisfying Dy Dx Y 2 2xy X 2 Y 2 2xy X 2 And Passing Through 1 1 Is




For The Differential Equation X 2 Y 2 Dx 2xy Dy 0 Which Of The Following Are True A Solution Is X 2 Y 2 Cx B X 2 Y 2 Cx C X 2 Y 2 X C D Y 0 0
For example, we can use this substitution $u=xy$, $v=x^2y^2$ $$ (x^22xyy^2)dy (x^22xyy^2)dx = \\ (x^2y^2)(dxdy)2x^2dx2y^2dy2xydx2xydy=\\ (x^2y^2)(dxdy)(xy)(2xdx2ydy)=\\ vduudv=0 $$ Thus we conclude that $v=Cu$ , and we can find $y(x)$ from this Solve differential equations (x 2y 2 − 1)dy 2xy 3dx = 0 We now what ∂M ∂y = 2x2y and ∂N ∂x = 2y3 I try to make it exact but get this x2 − y2 xy2 Help me!Simple and best practice solution for (3x^22xy2x)dx(x^22y)dy=0 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve it



1




Y 2xy Dx 2x 3xy Dy 0 Please Solve Edurev Physics Question
This is almost certainly a problem in a section of the text on "exact" equations, ie ones of the form dF= F,x dx F,y dy =0 The solution is F(x,y)=constant You need to look for an integrating factor A such that F,x = A 2 x y F,y = A (x^2 y^2Math\text{Use a substitution} \left\{\begin{array}{l} u = \frac{y}{x} \\ y = ux \\ \mathrm dy = u \,\mathrm dx x \,\mathrm du \end{array}\right/math math(x 3 Q1B Solve (1) Two circles with radii 16 and 48 touch each other externally Find the distance between their centres
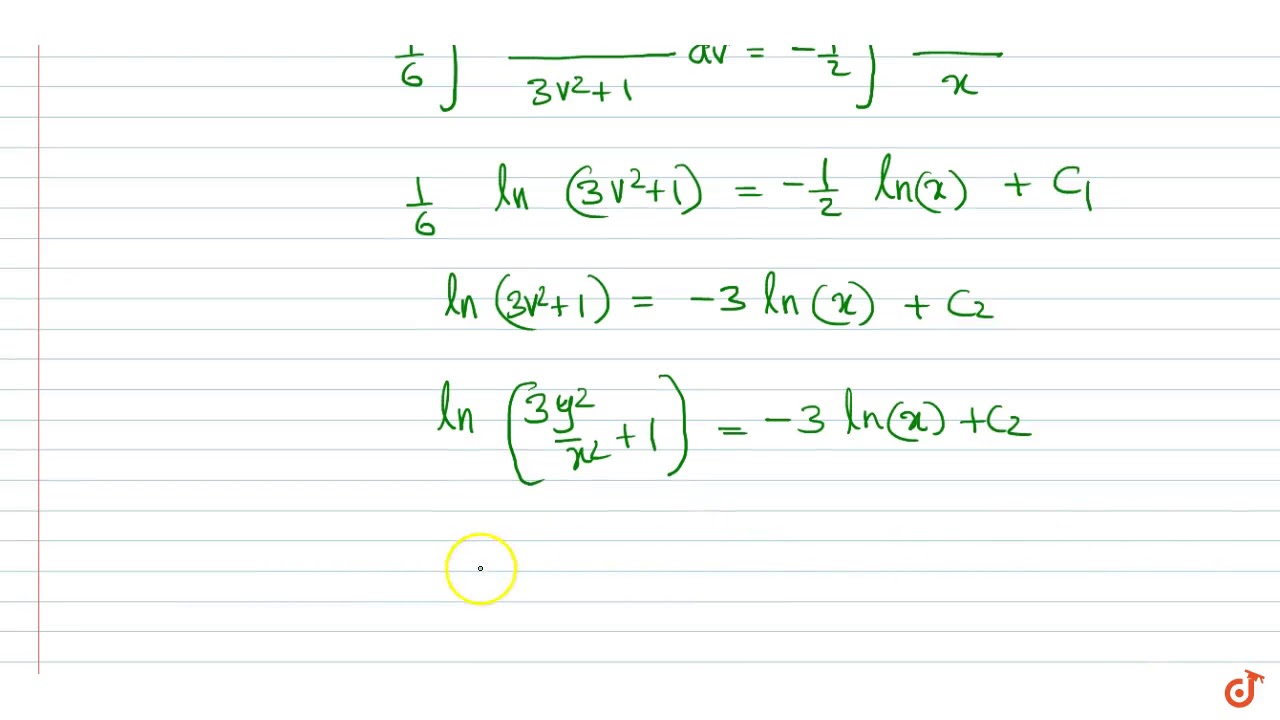



Solve The Differential Equation X 2 Y 2 Dx 2xydy 0 Youtube




How Does 2xydx Y 2 X 2 Dy 0 Quora
The ODE is homogeneous ODE of order one This is because the coefficients of dx and dy are both homogeneous two variables functions of the same order I suggest you write the ODE as y′ = 32t2t2−t−2 = f (t), (x = 0,t = y/x) Find the solution of (xy^22x^2y^3)dx (x^2yx^3y^2)dy=0 In differential equation show that it is homogeneous and solve it dy/dx = x^2 y^2/2xy asked Aug 9 in Differential Equations by Devakumari ( 192k points) differential equationsCombine all terms containing d \left (y^ {2}xyx^ {2}\right)d=0 ( y 2 x − y x 2) d = 0 The equation is in standard form The equation is in standard form \left (xy^ {2}yx^ {2}\right)d=0 ( x y 2 − y x 2) d = 0 Divide 0 by y^ {2}xyx^ {2} Divide 0 by y 2 x − y x 2




Solved Problems In Differential Equations 2500 Solved Problems In Differential Docsity




Consider The Equation Y 2 2xy Dx X 2 Dy 0 Chegg Com
(y^22xy)dx (x^2) dy = 0 Integrating factor y^2 solution xx^2/y = C Finding the integrating factor Giving these things some names names M(x,y) = y^22xy N(x,y) = x^2 M(x,y) dx N(x,y) = 0 We're looking to make this an exact equation, because if we do, it can be solved rather systematically In order to be exact, by claurait's theoem (think that's the name of Ex 95, 4 show that the given differential equation is homogeneous and solve each of them (𝑥^2−𝑦^2 )𝑑𝑥2𝑥𝑦 𝑑𝑦=0 Step 1 Find 𝑑𝑦/𝑑𝑥 (𝑥^2−𝑦^2 )𝑑𝑥2𝑥𝑦 𝑑𝑦=0 2xy dy = − (𝑥^2−𝑦^2 ) dx 2xy dy = (𝑦^2−𝑥^2 ) dx 𝑑𝑦/𝑑𝑥 = (𝑦^2 − 𝑥^2)/2𝑥𝑦 Step 2 Putting F(x, y) = 𝑑𝑦/𝑑𝑥 and finding FSolutionShow Solution (x 2 y 2 )dx 2xy dy = 0 ∴ 2xy dy = (x 2 y 2 )dx ∴ `"dy"/"dx" = ("x"^2 "y"^2)/"2xy"` (1) Put y = vx ∴ `"dy"/"dx" = "v x"du"/"dx"` ∴ (1) becomes, v x`"du"/"dx" = ("x"^2 "v"^2"x"^2)/ ("2x" ("vx"))` ∴ `"v x""du"/"dx" = (1 "v"^2




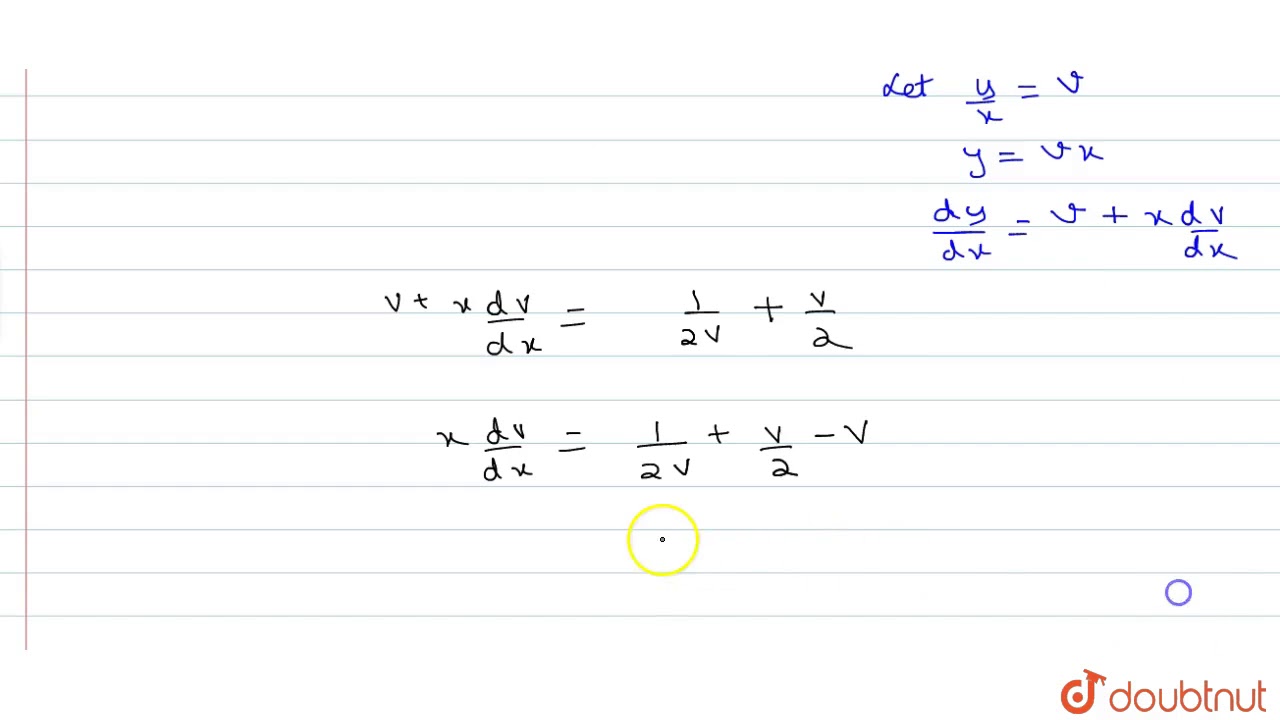
Dx V Tv Y2 2xy Dx X2 2xy Dy Theo



How To Solve The Homogeneous First Order Equation X 2 Y 2 Dx 2xydy 0 Quora
`dy/dx=(y^22xy)/x^2` `=y^2/x^2(2xy)/x^2` `=(y/x)^22(y/x)` Now let v = y/x Then y = vx and y' = v'xv `dy/dx=v^22v=(dv)/dx*xv` `v^2v=x(dv)/dx` `1/xdx=1/(v^2v)dv` `lnx =Solution for (2xy)dy (x^2y^21)dx=0 equation Simplifying (2xy) * dy 1 (x 2 y 2 1) * dx = 0 Remove parenthesis around (2xy) 2xy * dy 1 (x 2 y 2 1) * dx = 0 Multiply xy * dy 2dxy 2 1 (x 2 y 2 1) * dx = 0 Reorder the terms 2dxy 2 1 (1 x 2 y 2) * dx = 0 Reorder the terms for easier multiplication 2dxy 2 1dx (1 x 2 y 2) = 0 2dxy 2 (1 * 1dx x 2 * 1dx y 2 * 1dx) = 0 Reorder the terms 2dxy 2 (1dx 1dxy 2 1dx 3) = 0 2dxy 2 (1dx 1dxy 2 If the curve, y = y(x) represented by the solution of the differential equation (2xy^2 y)dx xdy = 0, asked Mar 10 in Mathematics by Takshii ( 347k points) jee




4 A Solve The Differential Equation 2xy Dy Dx Gauthmath




4 Solve The Exact Differential Equation 1 2xy Dx 4y3 X2 Dy 0 4 Solve The Exact Differential Equation Homeworklib
How do you use Implicit differentiation find #x^2 2xy y^2 x=2# and to find an equation of the tangent line to the curve, at the point (1,2)?Calculus Find dy/dx x^2y^2=2xy x2 y2 = 2xy x 2 y 2 = 2 x y Differentiate both sides of the equation d dx (x2 y2) = d dx (2xy) d d x ( x 2 y 2) = d d x ( 2 x y) Differentiate the left side of the equation Tap for more steps Differentiate Tap for more stepsThe solution of the differential equation dy / dx = xy y / xy x is The solution of the differential equation x (dy / dx) 2y = x 2 is The solution of the equation (3 2 √2) x^28 (3 2 √2) 8x^2 = 6 are The Solution Of The Equation Sin X Cos X Power 1 Sin 2x 2 Pi X Pi Is The Solution Of Trigonometric Equation Cos 4 X Sin 4



3x 2 2xy 2 Dx 6y 2 X 2 3 Dy 0 Sarthaks Econnect Largest Online Education Community
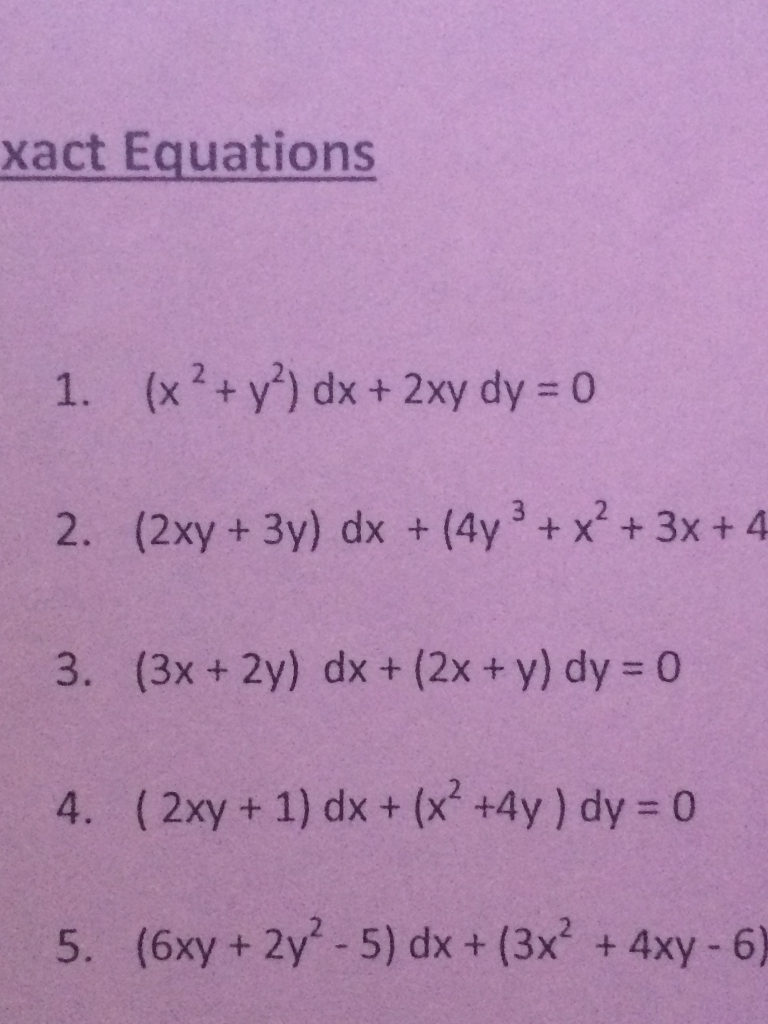



Xact Equations 1 X2 Y2 Dx 2xy Dy 0 2 2xy 3y Chegg Com
I'm at the beggining of a differential equations course, and I'm stuck solving this equation $$(x^2y^2)dx2xy\ dy=0$$ I'm asked to solve it using 2 different methods I proved I can find integrating factors of type $\mu_1(x)$ and $\mu_2(y/x)$If I'm not wrong, these two integrating factors are $$\mu_1(x)=x^{2} \ \ , \ \ \mu_2(y/x)=\left(1\frac{y^2}{x^2}\right)^{2 Solved Solve differential equation 1y^2xy^2)dx(x^2yy2xy)dy=0 First Order Bernoulli differential equation is of the form \(y'p(x)y=q(x)y^n\) \((1y^2xy^2)(x Stack Exchange network consists of 178 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange




Exact Differential Equations




9 Solve Dy Dx 2xy X 2 Y 2 10 Solve 2xydx 1 Chegg Com
2xy(dy divide by dx) equally x squared plus 2y squared ;The given vector field is math(x^22xy)\hat i(x^23)\hat j/math We want to compute its line integral along mathy^2=8x/math and mathx=2,/math by line integral method as well as by Green's Theorem Line integral method The path consisSolution for Solve dy/dx=2xy/(x^2y^2) Q A group of 150 tourists planned to visit East AfricaAmong them, 3 fall ill and did not come, of th A Consider the provided question, First draw the Venn diagram according to the given question, Let K r




First Order Differential First Order Differential Equations 3 3 X2 Y2 Dx 2xy Dy 0 Dy Dx Y2 X2 2xy Pdf Document



X Y 2 Dx 2xy Dy 0 Sarthaks Econnect Largest Online Education Community
Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreThe solution of differential equation (x 2 y – 2xy 2) dx – (x 3 – 3x 2 y) dy = 0, is SOLUTION Concept If an equation of the form M dx N dy = 0 is inexact, it is made exact by multiplying integrating factor IFQuestion dy = x2 (Sin2x 2xy) dx y(1) = 2 calculate the values of y(x) when 1




X2 2xy Y2 Dx X Y 2 Dy 0
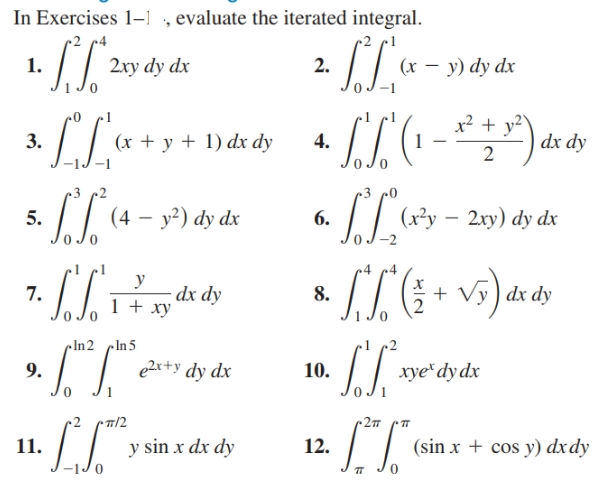



Answered In Exercises 1 1 Evaluate The Bartleby
Click here👆to get an answer to your question ️ Solve ( x^2 y^2 ) dy/dx = 2xy Join / Login > 12th > Maths > Differential Equations > Solving Homogeneous Differential Equation Solve the differential equation d x d y = x 3 3 x y 2 y 3 3 x 2 y (3y^22xy)dx (2xyx^2)dy=0 classify the equation linear, nonlinear, separable,exact, homogeneous, or one that requires an integration factor' and find homework help for other Math questions at Ex 95, 15 For each of the differential equations in Exercises from 11 to 15 , find the particular solution satisfying the given condition 2𝑥𝑦𝑦^2−2𝑥^2 𝑑𝑦/𝑑𝑥=0;𝑦=2 When 𝑥=1 Differential equation can be written 𝑎s 2𝑥𝑦𝑦^2−2𝑥^2 𝑑𝑦/𝑑𝑥=0 2𝑥𝑦𝑦^2= 2𝑥^2 𝑑𝑦/𝑑𝑥 2𝑥^2 𝑑𝑦/𝑑𝑥=2𝑥𝑦𝑦^2 𝑑𝑦/




Y 2 2xy Dx X 2 2xy Dy




Ex 9 5 15 Class 12 Find Solution 2xy Y 2 2x 2 Dy Dx 0 When
This is a homogeneous differential equation because it has homogeneous functions of same degree 2 homogeneous functions are (x2 y2) and 2xy, both functions have degree 2 Solution of differential equation Equation (i) can be written as, dy dx = 1 y x 2 ( y x) (ii) Substitute, y x = v dy dx = xdv dx v0 votes 1 answer The general solution of the DE dy/dx y/x x^2 is$$ x^{2} 2 x y{\left(x \right)} \frac{d}{d x} y{\left(x \right)} 2 y^{2}{\left(x \right)} = 0$$ Do replacement




Exact Equations Example 3 Video Khan Academy



How To Integrate X Y 2 Dx 2xy X 2 Y Dy 0 Quora
Solve the differential equation (x^2 y^2)dy/dx = 2xy given that y = 1, x = 1 asked in Differential equations by AmanYadav ( 556k points) differential equationsGiven diffequ is dy/dx= (y^2–2xy)/ (x^2–2xy) Take y=ux so that ux du/dx= (u^2–2u)/ (1–2u) ie du/dx=3 (u^2u)) (1–2u) ie ((1–2u)/ (uu^2)) du= (—3/x)dx Integrating we get log (uu^2)=3logxlogc Hence x^2yy^2x=c is the required general solutionFind dy/dx 2xyy^2=1 Differentiate both sides of the equation Differentiate the left side of the equation Tap for more steps By the Sum Rule, the derivative of with respect to is Evaluate Tap for more steps Since is constant with respect to , the derivative of with respect to is



Http Fractal Math Unr Edu Ejolson 285 15 Extraprob Pdf




Solve Y 2 2x 2y Dx 2x 3 Xy Dy 0 Mathematics Stack Exchange




Y 2 2xy Dx X 2dy 0 Reshit Differencialnoe Uravnenie



Solved Solve The Following Ordinary Differential Equation X2 Y2 Dx 2xy Dy 0 Find A Vector That Is Orthogonal To The Plane Containing The Points Course Hero




Vector Analysis By Alimkanwalimtinaa Issuu
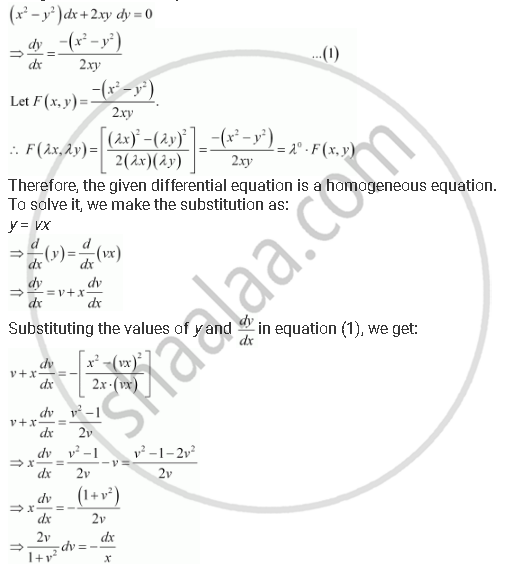



Show That The Given Differential Equation Is Homogeneous And Solve Each Of Them X2 Y2 Dx 2xy Dy 0 Mathematics Shaalaa Com
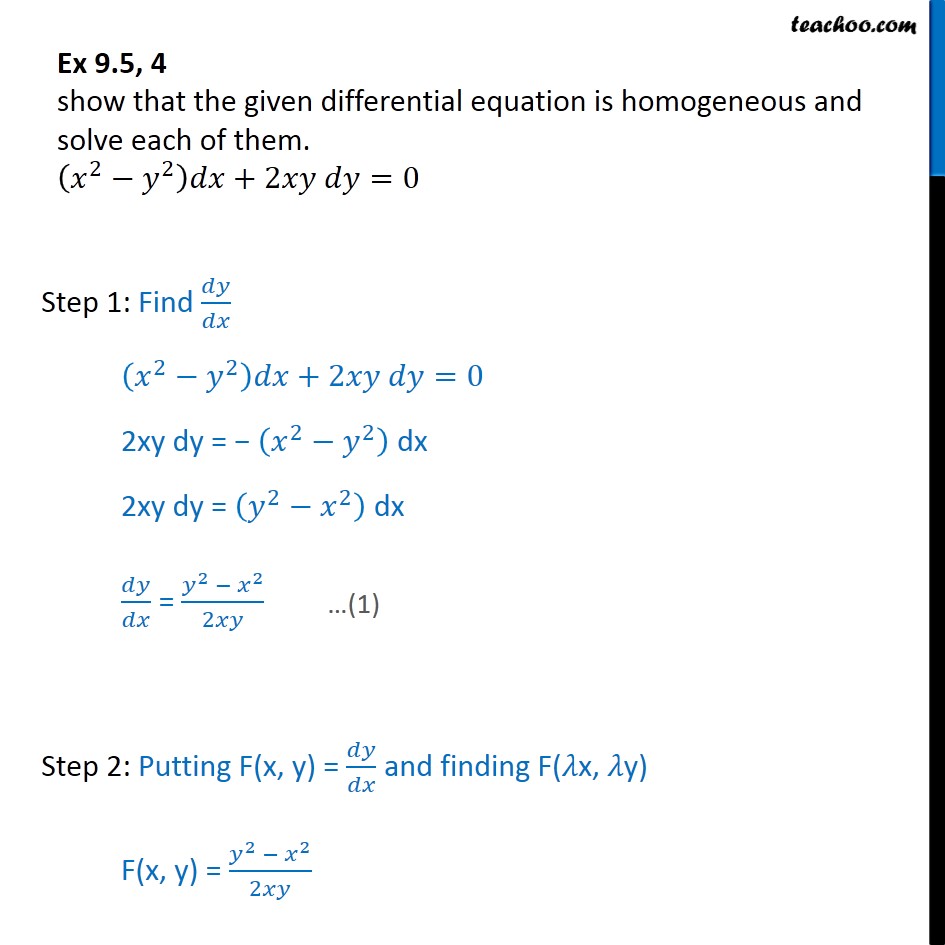



Ex 9 5 4 Show Homogeneous X2 Y2 Dx 2xy Dy 0 Ex 9 5




Solving Differential Equation 2xy Dy Dx Y 2 X 2 0 Youtube




For The Differential Equations Find The Particular Solution Satisfying The Given Condition 2xy Y 2 2x 2 Dy Dx 0 Y 2 When X 1 Mathematics Shaalaa Com




X 2 Y 2 Dx 2xy Dy 0 The Solution To This Differential Equation Represents Which Curve




Differential Equations Solved Examples Show That Following Differential Equation Is Not Exact 3x 2y 4 2xy Dx 2x 3y 3 X 2 Dy 0 Then Find An Integrating Factor To Solve The Differential Equation



Answered Rebecca Ferrer 2 26 Given Bartleby



1



Solve The Following Differential Equation 2x 2 Dy Dx 2xy Y 2 0 Sarthaks Econnect Largest Online Education Community



What Is The Solution Of Math Frac Dy Dx Frac 2xy X 2 Y 2 Math Quora
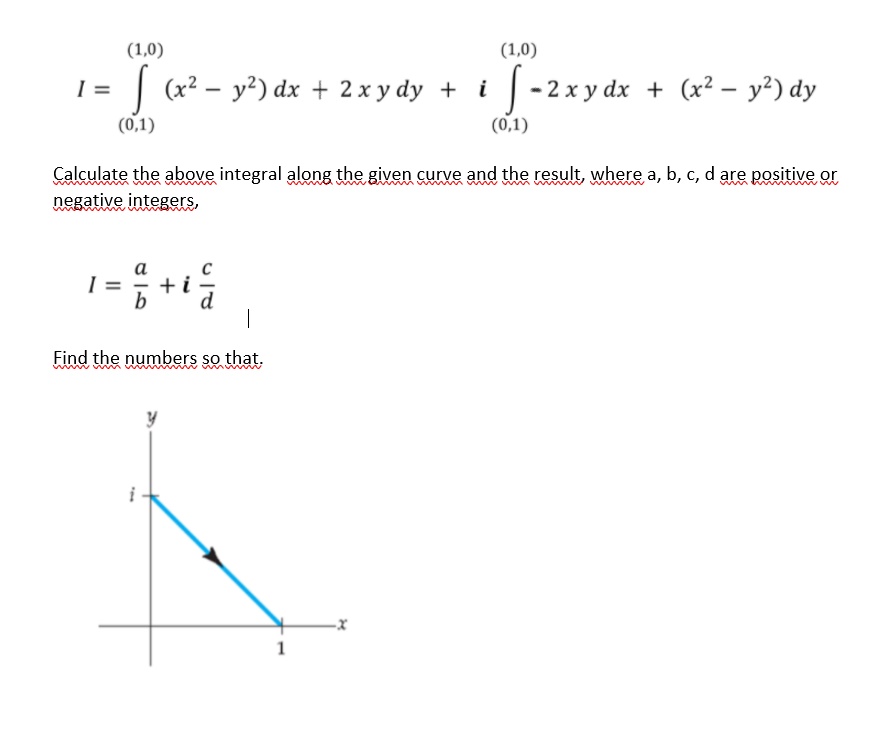



Answered 1 0 1 0 X Y Dx 2xy Dy Bartleby




Engineering Mathematics Notes
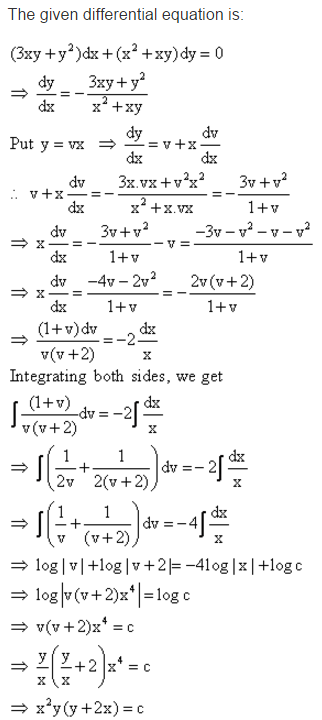



Find The Particular Solution Of Given Differential Equation 3xy Y 2 Dx X 2 Xy Dy 0 At X 1 Y 1 Mathematics Topperlearning Com D1ksg633




Solve The Differential Equation 1 X2 Dy 2xy Dx Cot X Dx X Is Not Equal To 0 Maths Differential Equations Meritnation Com




Y4 2xy Dx 3x2 Dy 0 When X 2 Y 1 Wegglab
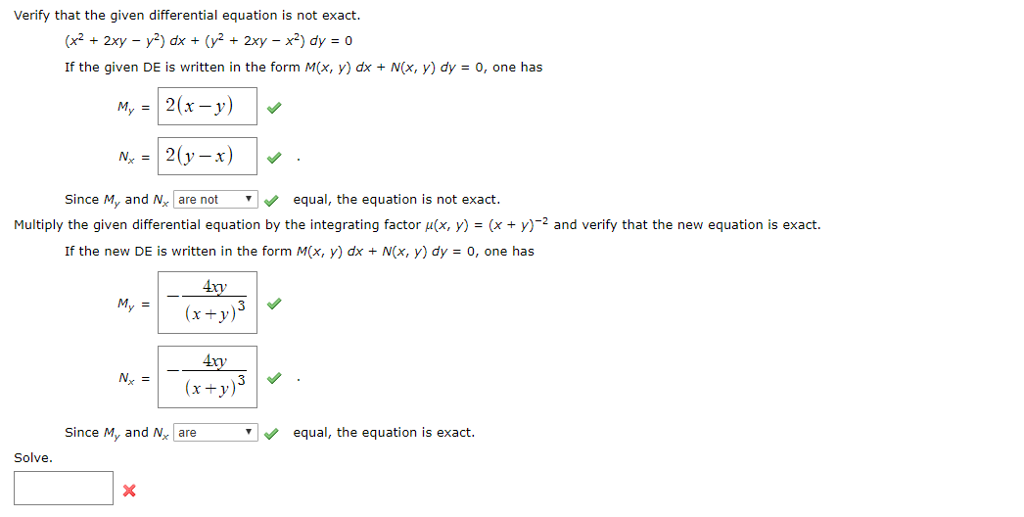



Verify That The Given Differential Equation Is Not Chegg Com



Solve The Following Differential Equation X2 Y2 Dx 2xy Dy 0 Given That Y 1 When X 1 Or Solve The Following Differential Equation If Y 1 When X 1 Mathematics Topperlearning Com 237hoo
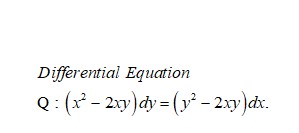



Answered Differential Equation Q X 2xy Dy Bartleby




X2 2xy Y2 Dx X Y 2dy 0 Brainly In




Solve Y 2 2x 2y Dx 2x 3 Xy Dy 0 Mathematics Stack Exchange
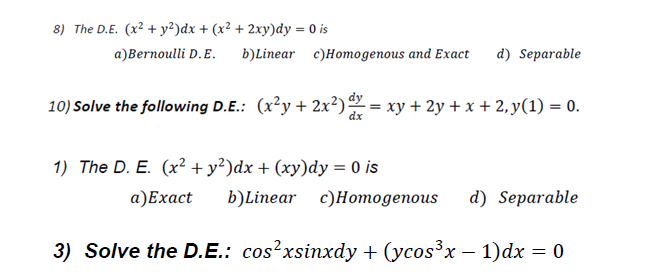



The D E X 2 Y 2 Dx X 2 2xy Dy 0 Is Chegg Com



Solved Solve The Following Ordinary Differential Equation X2 Y2 Dx 2xy Dy 0 Find A Vector That Is Orthogonal To The Plane Containing The Points Course Hero




15 2xy Y 2 Dx 2xy X 2 2x 2y 2 2xy 3 Dy 0 Homeworklib



X 2 Y 2 Dx 2xydy 0 Cloebeautifull



Solve The Differential Equation Dy Dx 2xy Y Studyrankersonline
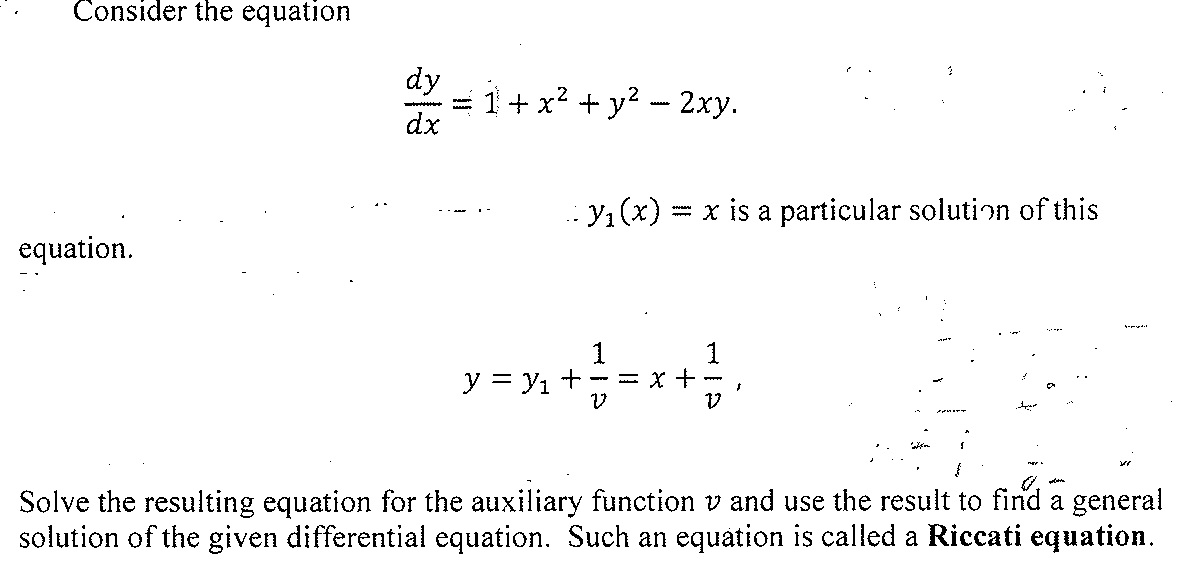



Consider The Equation Dy Dx 1 X 2 Y 2 2xy Chegg Com




In Solve The Given Initial Value Problem X Y 2 Chegg Com



X 2 Y 2 Dx 2xy Dy 0 Youtube




Solve X 2 2xy Y 2 1 Dy Dx 2 X Y



Solved Solve The Homogeneous Differential Equation X2 Y2 Dx 2xy Dy 0 Course Hero




Ex 9 6 14 Find Particular Solution 1 X2 Dy Dx 2xy




Solve Y 2 2xy Dx X 2 2xy Dy
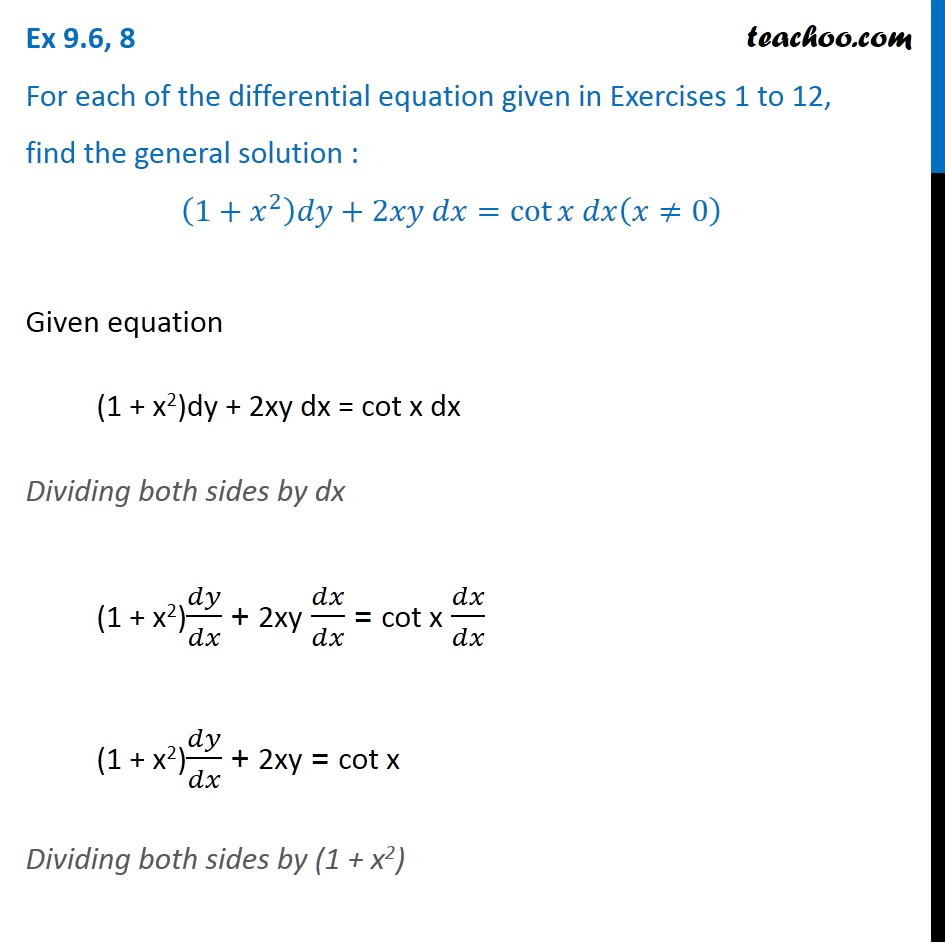



Ex 9 6 8 Find General Solution 1 X2 Dy 2xy Dx Ex 9 6



Ma2e01 Tutorial Solutions 8 1 2 R



How To Solve The Differential Equation X Y Dx 2xydy 0 Quora



How To Solve Show The Differential Equations Are Exact 2xy Y Tany Dx X 2 X Tany 2 Secy 2 2 Dy 0 Te Tx 2x Dx Dt Xe Xt 0 Quora




Kiyos06 3 0 Y 2 X 2 Dy Dx 2xy 0 3 1 2xy Dx Dy Y 2 X 2 3 2 Dx 2 Dlogy Y 2 X 2 3 3 X 2 W Logy Z 3 4 Dw Dz W E 2z 3 5 W Ce Z 1 3 E 2z 3 6 X 2 C Y 1 3 Y 2 Differentialequation Differential Equation




Solve Dy Dx 2xy X2 Y2 The Answer Given In The Book Is Y C X2 Y2 Brainly In




Verify That The Given Differential Equation Is Not Chegg Com




15 The Differential Equation 2xy Dy X2 Y2 1 Dx Determines A A Family Of Circles With Centre On X Axis B A Family Of Circles With Centre On Y Axis



Solve The Differential Equation X2 1 Dy Dx 2xy 1 X2 1 Studyrankersonline




Engineering Mathematics Notes



Solve X 2 Y 2 Dx 2xy Dy 0 Sarthaks Econnect Largest Online Education Community




Show That The Equation 5x4 3x2y2 2xy3 Dx 2x3y 3xy2 5y4 Dy 0 Is An Exact Differential Equation Find Its Solution Mathematics 2 Question Answer Collection



1




7 X 2 Y 2 Dx X 2 2xy Dy 0 Ecuacion Diferencial Exacta Youtube




2 X 2 3xy Dy Y 2 2xy Dx 0 Brainly In



For The Differential Equation X 2 Y 2 Dx 2xy Dy 0 Which Of The Following Are True Youtube



How To Solve Show The Differential Equations Are Exact 2xy Y Tany Dx X 2 X Tany 2 Secy 2 2 Dy 0 Te Tx 2x Dx Dt Xe Xt 0 Quora



2




What Is The Solution To This Ode 3x 2 4xy Y 2 Dx 2x 2 2xy 9 Dy 0 Quora




Find A One Parameter Family Of Solutions Of Each Of The Following Differential Equation Y2 2xy Dx X2 2xy Dy Own Classes




Pure Mathematics Find Dy Dx In Terms Of X And Y When X Y 2xy 3y 2x 7 S O L U T I O N 1 This Is An Implicit Function Because We




How To Find Exact Equation Quick And Easy Differential Equations Explained Right Youtube




Dx Dt 4x X 2 2xy Dy Dt Y 0 5 Xy A Find Equilibrium Points B Find Jacobian Matrix For Above System C Find Jacobian Matrix At Eq Point 0 0 D Draw Phase Portrait



Solve The Following Differential Equation 1 X 2 Dy Dx 2xy 1 1 X 2 Given Y 0 When X 1 Sarthaks Econnect Largest Online Education Community



X 2 Y 2 Dx Xydy 0 Happylove22



Www Tau Ac Il Levant Ode Solution 6 Pdf




12th Std Maths Ex 10 6 5 Solve The Differential Equation Y 2 2xy Dx X 2 2xy Dy Apho18



Solve Dy Dx X 2 3y 2 2xy Youtube




First Order Differential Equations Ppt Download
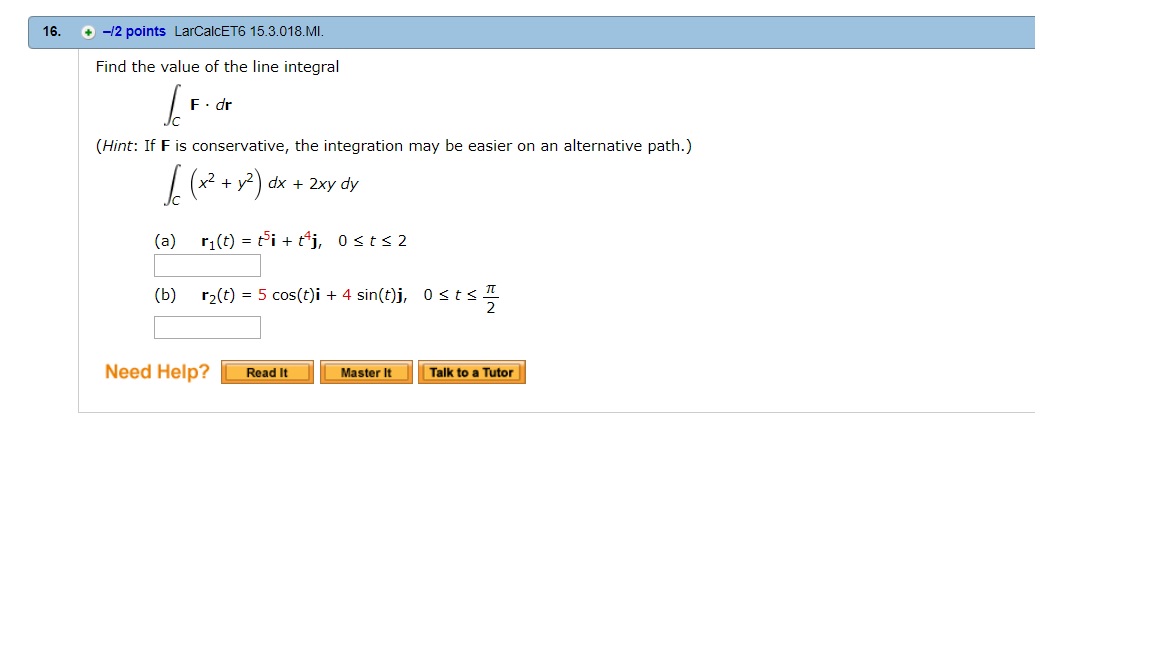



Find The Value Of The Line Integral F Dr C Hint If F Is Conservative The Integration May Be Easier On An Alternative Path X2 Y2 Dx 2xy Dy Socratic



Pmt Physicsandmathstutor Com Download Maths A Level C4 Topic Qs Edexcel Set 1 C4 differentiation implicit differentiation Pdf




1 X 2 Dy Dx 2xy X 2 2 X 2 1 Youtube




Y 2e X 2xy Dx X 2dy 0 Youtube




2xy Dy Dx X 2 3y 2 Youtube



Http Wwwf Imperial Ac Uk Jdg Ee22soln Pdf



How To Solve X 1 Dy 2xy Dx 0 Quora
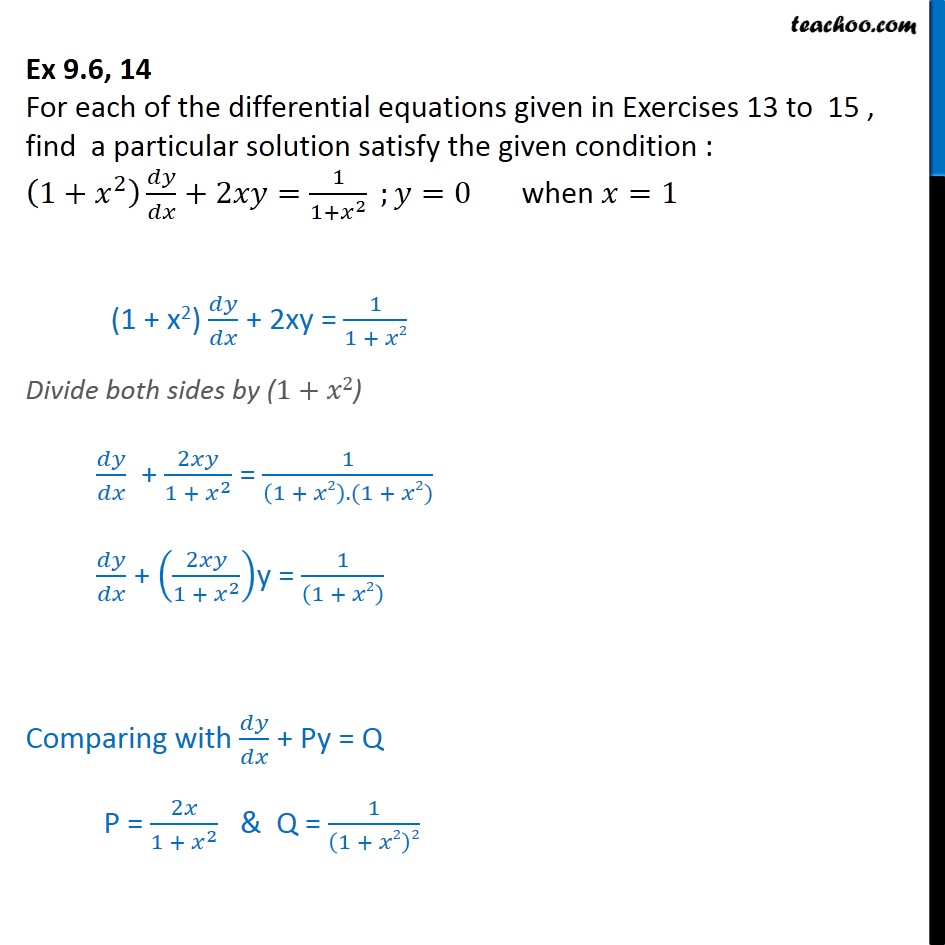



Ex 9 6 14 Find Particular Solution 1 X2 Dy Dx 2xy




1 Given The Differential Equation X2 2xy Y2 Dx Y Chegg Com




Find Dy Dx If X 2 2xy Y 2 0 Brainly In


